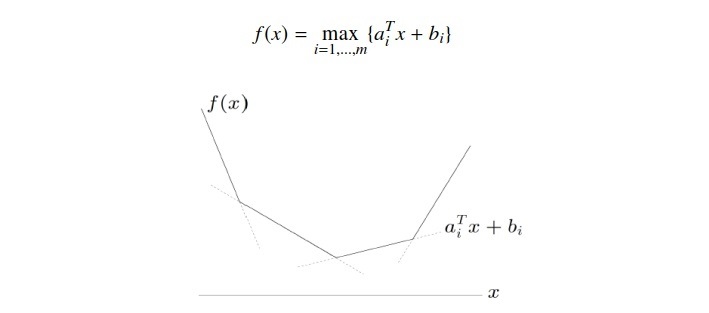
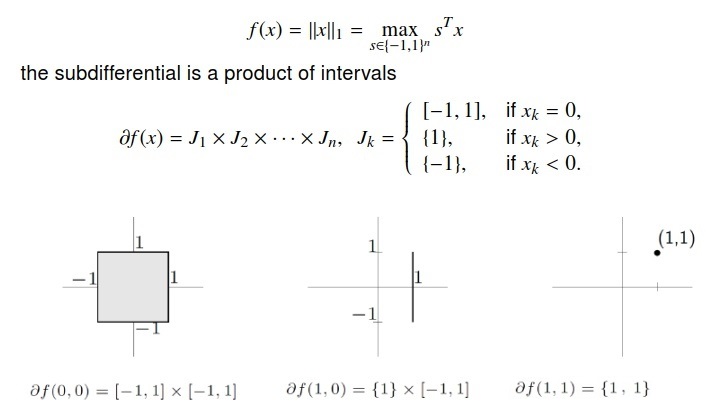
Cone

• 锥：$C \subset V, \forall x \in C, a>0 \Rightarrow ax \in C$

  • 锥总是无界的

  • $V$：向量空间

• Convex Cone 凸锥：$\forall x,y \in C, \forall a,b > 0 \Rightarrow ax + by \in C$

  • 凸锥必然是凸集
  • 非凸锥：凸锥的补集

• Norm Cone $n$ 维标准锥：$C = { (x,t)| |x|_2 \leq t, x \in R^{n-1}, t \in R }$

• Second Order Cone 二阶锥：$C = { (x,t)|Ax+b|_2 \leq c^Tx + d }$

  • 二阶锥相对于对标准锥做了仿射变换（平移变换）

Notations and Terminology

Strong Convexity

• 凸函数 $f$ 满足

  $$\forall x, y \in R, \forall \lambda \in (0,1), f(\lambda x + (1-\lambda) y) \leq \lambda f(x) + (1-\lambda)f(y)$$

• 强凸函数：不等式严格不等的凸函数

  • 为保证强凸性，常添加二次项保证，如：增广拉格朗日

凸集相关标记

• $C \subseteq R^N$：非空凸集
• $x \in R^N$

• 点 $x \in R^N$ 到 $C$ 的距离为

  $$D_C(x) = \min_{y \in C} \|x-y\|_2$$

• 点 $x \in R^N$ 在 $C$ 上投影为

  $$P_Cx \in C, D_C(x) = \|x - P_Cx\|_2$$
  • $C \subseteq R^N$：闭凸集

• Indicator Function 凸集 $C$ 的示性函数为

  $$l_C(x) = \left \{ \begin{array} 0 & if x \in C \\ +\infty & if x \notin C \end{array} \right.$$

齐次函数

齐次函数：有倍数性质的函数，若变量乘以系数 $\alpha$，则新函数为原函数乘上 $\alpha^k$ 倍

$$f(\alpha x) = \alpha^k f(x)$$

• $\alpha \neq 0 \in F, x \in X$
• $f: X \rightarrow W$：域 $F$ 内两个向量空间之间的 $k$ 次齐次函数

• 线性函数 $f: X \rightarrow W$ 是一次齐次函数
• 多线性函数 $f: x_1 x_2 \cdots * x_n \rightarrow W$ 是 $n$ 次齐次函数

基本定理

• 欧拉定理：函数 $f: R^n \rightarrow R$ 可导、$k$ 次齐次函数，则有 $x \nabla f(x) = kf(x)$

次梯度

• 次梯度：实变量凸函数 $f$ 在点 $x_0$ 的次梯度 $c$ 满足

  $$\forall x, f(x) - f(x_0) \geq c(x - x_0)$$

• 可证明凸函数 $f$ 在 $x_0$ 处所有次梯度的集合 $\partial f(x)$ 是非空凸紧集

  • $\partial f(x) = [a, b]$，其中$a, b$为单侧极限

    $$\begin{align*} a & = lim_{x \rightarrow x_0^{-}} \frac {f(x) - f_0(x)} {x - x_0} \\ b & = lim_{x \rightarrow x_0^{+}} \frac {f(x) - f_0(x)} {x - x_0} \end{align*}$$

• 凸函数均指下凸函数，梯度不减

次梯度性质

运算性质

• 数乘性质

  $$\partial(\alpha f)(x) = \alpha \partial f(x), \alpha > 0$$

• 加法性质

  $$\begin{align*} f &= f_1 + f_2 + \cdots + f_m, \\ \partial f &= \partial f_1 + \cdots + \partial f_m \end{align*}$$

• 仿射性质：$f$为凸函数

  $$\begin{align*} h(x) &= f(Ax + b) \\ \partial h(x) &= A^T \partial f(Ax + b) \end{align*}$$

最优化性质

• 凸函数 $f$ 在点 $x_0$ 可导，当且仅当次梯度仅包含一个点，即该点导数

• 点 $x_0$ 是凸函数 $f$ 最小值，当且仅当次微分中包含 0 （此性质为“可导函数极小点导数为 0 ”推广）

• 负次梯度方向不一定是下降方向

次梯度求解

• 逐点（分段）极值的函数求次梯度
• 求出该点相应极值函数
• 求出对应梯度即为次梯度

Pointwise Maximum

逐点最大函数：目标函数为

$$\begin{align*} f(x) & = max \{f_1(x), f_2(x), \cdots, f_m(x)\} \\ I(x) & = \{i | f_i(x) = f(x)\} \end{align*}$$

• $I(x)$：保存 $x$ 处取值最大的函数下标

• 弱结果：$I(x)$ 中随机抽取，以 $f_i(x)$ 在该处梯度作为次梯度

• 强结果

  $$\partial f(x) = conv \cup_{i \in I(x)} \partial f_i(x)$$
  • 先求支撑平面，再求所有支撑平面的凸包
  • 可导情况实际上是不可导的特殊情况

分段函数

• 折点处

  $$\partial f(x) = conv\{a_i, a_{i+1}\} = [a_i, a_{i+1}]$$

• 非折点处

  $$\partial f(x) = {a_i}$$

$L_1$范数

PointWise Supremum

逐点上确界：目标函数为

$$\begin{align*} f(x) &= \sup_{\alpha \in A} f_{\alpha}(x) \\ I(x) &= \{\alpha \in A | f_{\alpha}(x) = f(x)\} \end{align*}$$

• 弱结果：可行梯度为

  $$\partial (\max_{\alpha} f_{\alpha}(x)) \in partial f(x)$$

• 强结果

  $$\partial f(x) = conv \cup_{\alpha \in I(x)} \partial f_{alpha}(x) \subseteq \partial f(x)$$

最大特征值

$$\begin{align*} f(x) & = \lambda_{max}(A(x)) = \sup_{\|y\|_2 = 1} \\ A(x) & = A_0 + x_1 A_1 + \cdots + x_n A_n \end{align*}$$

• $A_n$：对称矩阵

• 对确定 $\hat {x}$，$A(x)$ 最大特征值 $\lambda_{max}$、对应特征向量 $y$，则该点此梯度为

  $$(y^T A_0 y, \cdots, y^T A_n y)$$

Pointwise Inferior

逐点下确界：目标函数为

$$f(x) = \inf_y h(x, y)$$

• $h$：凸函数

• 弱结果：给定$x = \hat x$，可行次梯度为

  $$(\partial h(x, \hat y)|_{x=\hat x}, 0) \in \partial f(x)$$

复合函数

$$f(x) = h(f_1(x), \cdots, f_n(x))$$

• $h$：凸、不降函数
• $f_i$：凸函数

• 弱结果：给定$x = \hat x$，可行次梯度为

  $$g = z_1 g_1 + \cdots + z_k g_k \in \partial f(\hat x)$$

  • $z \in \partial h(f_1(\hat x), \cdots, f_k(\hat x))$
  • $g_i \in \partial f_i(\hat x)$

  • 证明

    $$\begin{align*} f(x) & \geq h(f_1(\hat x) + g_1^T(x - \hat x), \cdots, f_k(\hat x) + g_k^T(x - \hat x) \\ & \geq h(f_1(\hat x), \cdots, f_k(\hat x)) + z^T(g_1^T(x - \hat x), \cdots, g_k^T(x - \hat x)) \\ & = f(\hat x) + g^T(x - \hat x) \end{align*}$$

次梯度法

$$\begin{align*} x^{(k+1)} & = x^{(k)} + \alpha_k g^{(k)} \\ f_{best}^{(k+1)} & = min{f_{best}^{(k)}, f(x^{(k+1)})} \end{align*}$$

• $g^{(k)}$：函数$f$在$x^{(k)}$处次梯度

• 求解凸函数最优化的问题的一种迭代方法

• 相较于较内点法、牛顿法慢

  • 应用范围更广泛：能够用于不可微目标函数，目标函数可微时，无约束问题次梯度与梯度下降法有同样搜索方向
  • 空间复杂度更小
  • 可以和分解技术结合，得到简单分配算法
  • 通常不会产生稀疏解

步长选择

• 次梯度法选取步长方法很多，以下为保证收敛步长规则

• 恒定步长：$\alpha_k = \alpha$
• 恒定间隔：$\alpha_k = \gamma / |g^{(k)}|_2$
• 步长平方可加、步长不可加：$\sum{k=1}^{\infty} \alpha_k^2 < \infty, \sum{k=1}^{\infty} \alpha_k = \infty$
• 步长不可加、但递减：$lim_{k \rightarrow \infty} \alpha_k = 0$
• 间隔不可加、但递减：$lim_{k \rightarrow \gamma_k} \gamma_k = 0$